Let's prove that if \(k\) is an integer, then \(\gcd(k, k+1) = 1\). That is, any two consecutive integers are coprime.
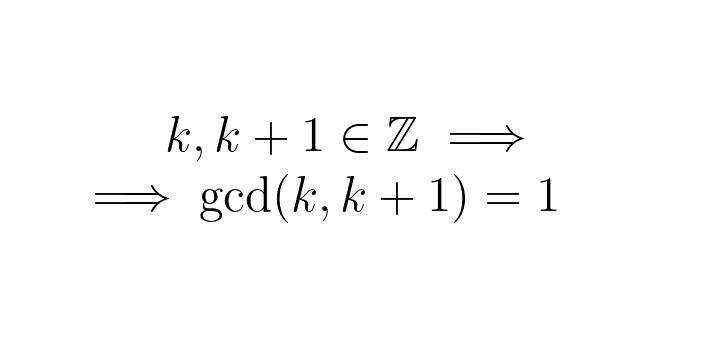
Twitter proof
Twitter proof:
— Mathspp (@mathsppblog) November 14, 2020
Let k be an integer and let d be the greatest common divisor of k and k+1. We have that (k+1)/d=k/d+1/d and both (k+1)/d and k/d are integers, so 1/d must be an integer and we can only have d=1.https://t.co/pItsAnueib
Do you have an idea for a twitter proof? Let me know in the comments below!
Become a better Python 🐍 developer 🚀
+35 chapters. +400 pages. Hundreds of examples. Over 30,000 readers!
My book “Pydon'ts” teaches you how to write elegant, expressive, and Pythonic code, to help you become a better developer. >>> Download it here 🐍🚀.