Let's prove that if you want to maximise \(ab\) with \(a + b\) equal to a constant value \(k\), then you want \(a = b = \frac{k}{2}\).

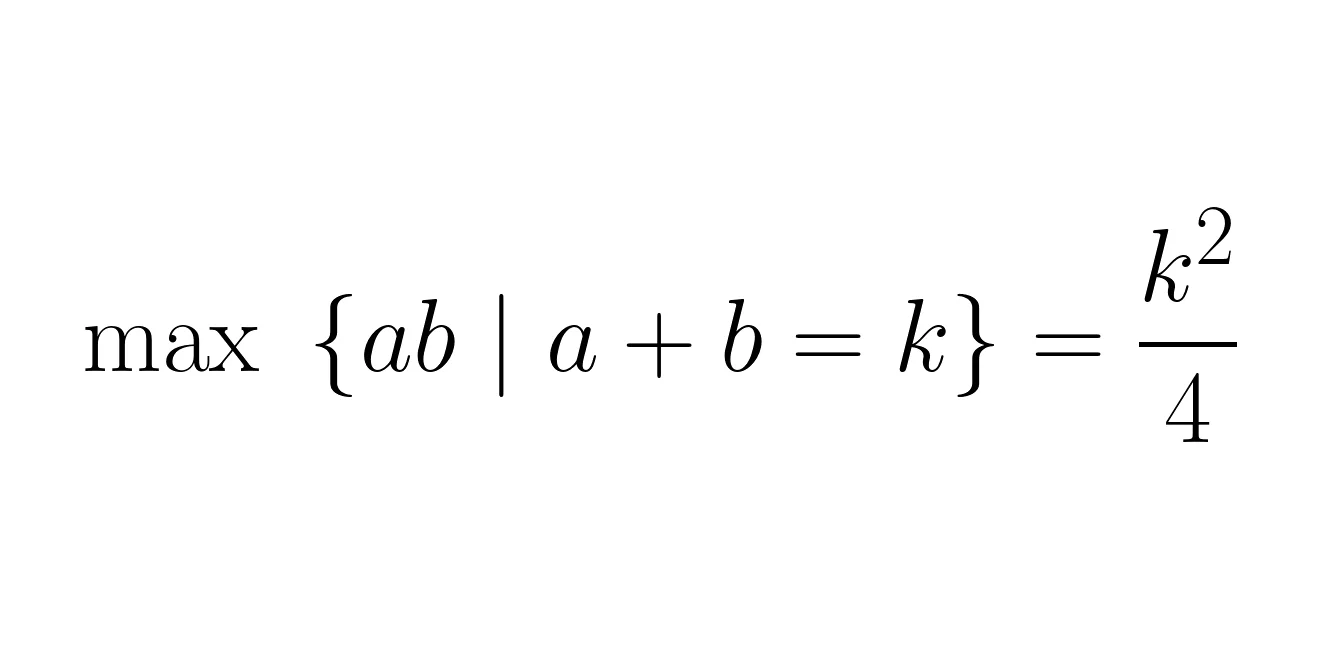
Twitter proof

Twitter proof:
Take s = k/2. If a = s+h then b = s-h, from which we get that ab = (s+h)(s-h) = s^2 - h^2. Because we know h^2 >= 0, ab is maximised when h = 0, that is a = b = s = k/2.https://t.co/9ypegSMF2F

— Mathspp (@mathsppblog) November 5, 2020

Do you have an idea for a twitter proof? Let me know in the comments below!

Thanks for reading this far! If you would like to show your appreciation for my work and would like to contribute to the development of this project, consider buying me a slice of pizza 🍕.